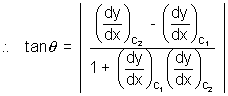application-of-derivatives-1

History of calculus is very old since 200 BC Bhaskara in 12^th century develop a no. of ideas that led to development of Rolle's Theorem. He was also first to define notion of derivative as a limit.

Leibniz & Newton pulled these ideas further. Newton was first to apply calculus to general physics. These great scholar developed fundamental theorem of calculus.

Today, calculus is used in every branching of physical sciences, in computer science, in statistics and in engineeerings, in economics, business etc.


Derivative as rate of change:

If variable quantity y is function of t i.e. y = f(t), then small change in time y in y
Average rate of change =
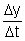
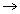
when t 0, rate of change becomes instantaneous
i.e.


Illustration: If radius of circle increasing at uniform rate of 2 cm/s, find rate of increasing of area of circle, at instant when radius is 20 cm.

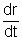
Ans:   = 2 cm/s
Area of circle = r²
Differentiating w.r.t. to t,

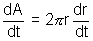
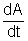
> = 2 x 20 x 2 = 80 cm²/s


Slopes of tangent & Normal:

Slope of tangent:


Let y = f(x) be cont. & P(x₁, y₁) be point on it.
Then is slope of tangent to curve y = f(x) at point P.

Note: (1) If tangent is 11 to x-axis, then
= 0

(2) If tangent is to x-axis, then
= 0


Dumb Question: How = 0 when tangent is 11 to x-axis.

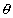
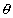
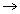
Ans: We know that slope of line = tan   where 0 with x-axis in anticlockwise direction.
If tangent is 11 to x-axis
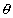
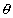
= 0   or   tan = 0



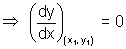Slope of Normal: Normal to curve at P is line to tangent at P & passing through P(x₁, y₁)
Slope of normal at P = -
                                

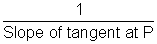
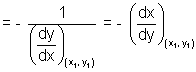Note: (i) If normal is 11 to x-axis


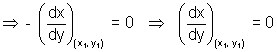
(ii) If normal is to x-axis
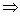
- = 0


Illustration: Find point on curve y = x³ - 3x at which tangent is 11 to x-axis.

Ans: Let the point be P(x₁, y₁)   on curve   y = x³ - 3x .................................... (i)
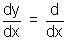
     (x³ - 3x) = 3x² - 3
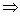
= 3x₁² - 3
But tangent is 11 to x-axis
  = 0
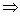
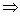
3x₁² - 3 = 0   x₁ = ±1 ........................................ (ii)
Since  P(x₁, y₁) lies on curve.

  y₁ = x₁³ - 3x₁

x₁ = 1                           where x₁ = - 1
y₁ = 1 - 3 = - 2              y₁ = - 1 + 3 = 2
  Points are (1, - 2) & (- 1, 2)


Equations of tangent & Normals:

Eq. of tangent:

Slope of tangent at P(x₁, y₁) = tan =
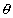
Since it passes through P(x₁, y₁)
eq. of tangent is
(y - y₁) = (x - x₁)


Eq. of Normal:

Slope of Normal = -
Eq. of normal is
(y - y₁) = - (x - x₁)


Illustration: Find eq. of tangent & normal to curve   2y = 3 - x² at (1,1)

Ans: Eq. of given curve is    2y = 3 - x² ...................................... (i)
differentiating (i) w.r.t.  x   
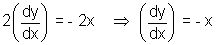
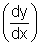_{(1, 1)} = - 1
eq. of tangent at (1, 1) is
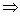
y - 1 = - 1(x - 1)   x + y = 2
& eq. of normal at (1,1) is
y - 1 = 1(x - 1) = y - x = 0


Angle of intersection of two curves:

It is angle b/w tangent s to the two curves at this point of intersection.


Let C₁ & C₂ be two curves of eq. is = f(x) & y = g(x) respectively.
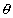
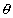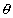Let is angle b/w two tangents of two curves & tangents PT₁ & PT₂ makes angle ₁ & ₂ respectively with x-axis.