application-of-derivatives-5

Properties of Monotonic function:

(1) If f(x) is strictly increasing function on [a, b]
{ f'(x) exists & f'(x) is also strictly increasing on [a, b].

(2) If f(x) & g(x) are also two continous & differentiable functions & fog(x) & gof(x) exists . then,

In other words
(i) If f(x) & g(x) are both strictly increasing or strictly decreasing.
(fog)(x) & (gof)(x) both are strictly inctreasing.

(ii) If amongst two functions f(x) & g(x), one is strictly increasing other is strictly decreasing.
(fog)(x) & (gof)(x) both are strictly decreasing.


Illustration: Let Ø(x) = sin(cosx) then check it whethe5r increasing ordecreasing in [0, ].

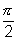
Ans: Ø(x) will be increasing if Ø'(x) > 0
Ø(x) = sin(cosx)
Ø'(x) = cos(cosx).(- sinx)
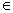
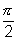
So, it is clearly decreasing for x [0, ] as Ø'(x) 0

Other method: f(x) = sinx & I(x) = cosx are increasing & decreasing in [0, ]
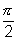
fog(x) = Ø(x) = sin(cosx) is decreasing.


Critical Points:

It is collection of points for which,
(i) f(x) does not exists.

(ii) f'(x) does not exists.

(iii) f'(x) = 0
All values of x obtained from above conditions are c/d critical points.


Illustration: Find critical points for f(x) = (x - 2)^2/3(2x + 1)

Ans: f(x) = (x - 2)^2/3(2x + 1)
f'(x) =
f'(x) =
Clearly f'(x) doesnot defined at x = 2 so, x = 2 is critical point.


Dumb Question: Why f'(x) is not defined at x = 2 ?

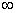
Ans: Putting x = 2, f'(x) = which is not defined.
Another critical point
f'(x) = 0
= 0   x = 1
So, x = 1 & x = 2 are two critical points of f(x)


Maxima & Minima:

Maxima: A function f(x) is said have maxima at x = a if f(a) f(a + h) where h 0 (very small & a lies in its domain.

Minima: A function f(x) is said to have minima at x = b if f(b) f(b + b) & f(b) f(b - b) where h 0 (very small)



Methods for finding extremum of continuone functions:

(a) First derivative test:

(i) When f(x) attains maximum at x = a:


From graph,
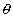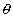For x < a, ₁ < 90⁰  tan₁ > 0 or increasing for x < a
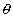
For x = a, tan = 0 or neither increasing nor decreasing for x = a.
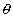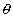For x > a, ₂ > 90⁰   tan₂ < 0 or decreasing for x > a
So,
f(x) is maximum at x =a


(ii) When f(x) attains minimum at x = b:


From graph,
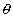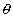For x < b, ₁ > 90⁰   tan₁ < 0 or decreasing when x - < b
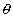
For x = b, tan = 0 or neither increasing nor decreasing for x = b
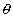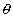For x > b, ₂ < 90⁰   tan₂ > 0 or increasing when x > b
So,
f(x) is minimum at x = b



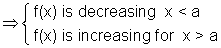Illustration: Let f(x) = x³ - 3x² + 6, find point at which f(x) have local maximum & local minimum.

Ans: f(x) = x³ - 3x² + 6
       f'(x) = 3x² - 6x
Critical points are if f'(x) = 0
    3x² - 6x = 0   x² - 2x = 0
x = 0, 2
At x = 0, f'(x) change sign from + ve to - ve & f'(x) changes sign from - ve to + ve at x = 2.
So, at x = 0, function has local maxima 7 at x = 2 it has local minima.


Second Derivative Test:

(1) First we find root of f'(x) = 0.
Let x = a is one roots of f'(x) = 0

(2) Now find f''(x) at x = a

(i) If f''(a) = -ve, then f(x) is max. at x = a.

(ii) If f''(a) = +ve, then f(x) is min. at x = a

(iii) If f''(a) = 0
Then find F'''(x) at x = 0
If f'''(a) = 0 then f(x) has neither maximum nor minimum at x = a.
But if f'''(a) = + ve, then find f'^v(a),
(a) If f'^v = + ve, then f(x) is min. at x = a.
(b) If f'^v(a) = - ve, then f(x) is max. at x = a.