Atomic Structure - 4

Size of nucleus:

Size of nucleus (r) = (1.3 x 10^-13) m^1/3
r 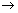rad. of nucleus     m 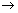Atomic no.


Dumb Question: Why radius of nucleus varies m^1/3 i.e. r 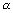m^1/3 ?

Ans: If nucleus isspherical,
density d =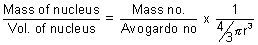
           d =
           r³ =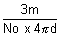
           r =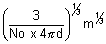
           r = 1.3 x 10^-13 x m^1/3
           r 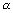m^1/3


……………………..


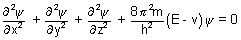
amplitude of wave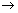
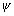
Coordinates of electron are (x, y, z), E is total energy of electron, v is its potential energy, m is mass of electron & h is planck’s constant.


Significance of wave function: Square of wave function (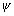) gives probability of finding electron at that point.
Probability of finding electron = 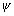²


Quantum No.: Set of foor no.s with help of which we can get complete information about all electrons in an atom i.e., location, energy, shap & orientation of orbital.

Principal quantam No. (n):

(i) It gives average distance of electron from nucleus.

(ii) It completely determines energy of electron in H-atom & H-like atoms.
       E_n = -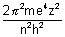
For I^st principal shell (k), n = 1 which means it has lowest energy & closest to nucleus.

(iii) Max. no. of elctrons present in any principal shell is given by 2n²   n 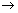no. of principal shell.


Question: ……………………

Ans: 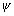is wave ………. schrodinger eq. It can be -ve or +ve but square of it always +ve & probability of finding electron at any place can not be -ve. So, it represents probability.

(i) It gives no. of subshells present in main shell.

(ii) Angular momentum of electron present in any sub shell.

(iii) Shapee of various subshells present within some principal shell.

Angular momentum of electron =
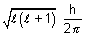
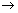Azimuthal quantum no.
For given value of n, it have integral value ranging from 0 to n - 1.
For 1^st shell, n = 1, = 0 & have only one subshell c/d s-subshell.
For II^nd shell, n = 2, = 0 & 1 & have two subshell s-subshell ( = 0)
& P-subshell ( = 1)
For III^rd shell, n = 3, = 0, 1, 2 & havethree subshell s, p & d - subshells. For d-subshell ( = 1).
For IV^th shell, n = 4, = 0, 1, 2, 3 & have 4 subshells s, p, d & f & for f subshell
( = 0)

Note: (1) No. of subshells present in any principal shell is equal to no. of principal quantum no.

(2) Energies of different subshells are found to be in order
       s < p < d < f


(1) It gives no. of orbitals present in any subshell.
For given , m has in5tegral value - to + .
For = 0, m has only one value i.e. m = 0
This means s-subshell has only one orientation in space.
For = 1, m has 3 value i.e.   m = - 1, 0 & + 1
This means p-subshell has 3 orbitals. These 3 orbitals are oriented along x-axis, y & z-axis. i.e. P_x, P_y & P_z.
For = 2, m have 5 values i.e. m = - 2, -1, 0, + 1 & + 2 d-subshell has 5 orbitals.
For = 3, m has 7 value, So, has 7 orbitals.

Note: By convention, for P_z, m = 0, & for P_x & P_y m ± 1 but not fixed.
Five d-orbitals. For dz², m = 0, for dxy & dx² - y², m = ± 1.


Degenerate orbitals: Those orbitals which have same subshell having equal energy.

(4) Spin Quantum No.:

(1) To explain magnetic properties of substance.

(2) Direction of electron spin i.e. clockwise or anticlockwise.
For any valve of m,   s = + 1/2   or   - 1/2


Illustration: An electron is in 4f orbital. What possible values for quantum numbers n, , m & s can it have ?

Ans: Since electron is in 4f orbital, value of principal quantum no., n = 4
For f-orbital, Azimuthal quantum no.   = 3. Values of magnetic quantum (m) are - to + in which zero.
       = 3, m = - 3, -2, -1, 0, +1, +2, +3
For each value of m, spin quantum no., s has two values, i.e., s = + 1/2 and s = - 1/2.


Pauli Exclusion Principle:

(1) No two electrons in an atom can have same set of four quantum numbers.
(2) An orbital can have maximum two electrons & these must have opposite spins.


Illustration: Let for 3s-orbital, It has n = 3, = 0 & m = 0. Since for each value of m, there are two values, spin quantum no. i.e. + 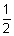and - 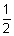. 3-s orbital can have two electrons; one with quantum no. n = 3, = 0, m = 0 & s = +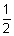
& other n = 3, = 0, m = 0 & s = -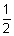
No. of orbitals in subshell = 2 + 1
Max. no. of electrons in subshell = 2(2 + 1)
No. of orbitals in n^th shell = n²
Max. no. of electrons in n^th shell = 2n²


Shape of s-orbitals

Shape of s-orbital is spherical.
For 1s, PRobability of electron is max. near nucleus & as distance .
For 2s, Prob. of electron is max. neaqr nucleus & then to zero & again & then as distance from nucleus .




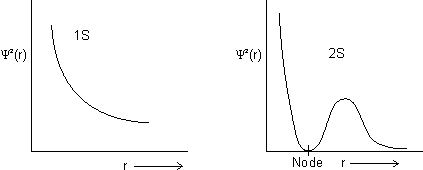
Dumb Question: What is node ?

Ans: It is region where probability of finding electron is zero c/d node.