Center of Mass -3

Motion of Center of Mass:

 

Differentiating with respect to time

Differentiating with respect to time

The Center of mass of the system is not affected by the internal forces. If the external forces add up to zero, then center of mass has no acceleration.

Consider a system of n particles. Now the i^th particle will be acted by 2 forces- internal forces due to rest of the (n-1) particles and the external forces. So far the i^th particle,

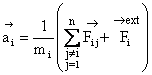
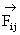= Force on i^th particle by j^th particle (j=i)

= Force on i^th particle by external forces.

Carrying summation over ‘i’ i.e. including all particles:

<!--[endif]--> <!--[if !mso]--> <!--[if !mso & !vml]-->

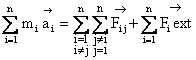
                        (Zero)

Dumb Question:

<!--[if !supportLists]--> 1)      Why =0?

Ans: The expansion will look like this:

Now

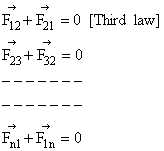
Now

Also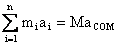

Hence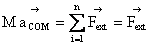

Hence if 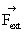=0 then 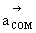=0.

Illustration:

Find the acceleration of center of mass?

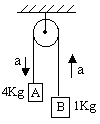
Assuming downwards as positive direction.

Illustration:

A projectile of mass 5m is projected from ground with velocity 50Ö2 at 45⁰ angle. At the highest point, it explodes into two pieces. One has a mass 4m while other has a mass m. Both pieces fly off in opposite horizontal direction. Mass 4m falls at a distance of 600m from point of projection. Find the distance of second mass from point of projection?

Solution:

Range of projectile otherwise;

So, if the distance of ‘m’ is x then,

Dumb Question:

<!--[if !supportLists]--> 1)      Why Center of mass follow same path?

Ans: Now if there had been no explosion, the projectile would have landed at 500m from point of projection. Now even if the projectile has exploded, the center of mass must follow same path as no extra force is acting.