b) Speed of Efflux:
Consider liquid of density r in a tank of large cross sectional area A1. There is a hole of cross-sectional area A2 at the bottom with liquid flowing out as shown in figure (7).
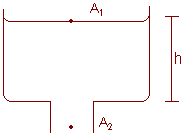
Fig (7)
Let V1 and V2 be the speed of the liquid at A1 and A2. Applying Bernoulli’s equation at A1 and A2 we have
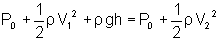
From equation of continuity A1V1 = A2V2
Solving above two equations we have
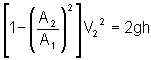
If A2<<A1, this equation reduces to
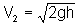
The speed of liquid coming out though a hole at a depth ‘h’ below the free surface is same as that of a particle fallen freely through the height ‘h’ under gravity. This is known as Torricelli’s theorem. The speed of the liquid coming out is called the speed of efflux.
c) Change of plane of motion of a spinning Ball.
Quite often when swing bowlers of cricket deliver the ball; the ball changes its plane of motion in air.
Such a deflection from the plane of projection may be explained on the basis of Bernoulli’s equation.
Suppose a ball spinning about the vertical direction is going ahead with some velocity in the horizontal direction in otherwise still air. Let us work in a frame in which the center of the ball is at rest. In this frame the air moves fast the ball at a speed V in the opposite direction. The situation is shown in Figure (8).
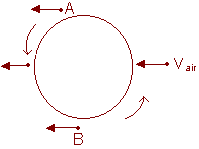
Fig (8)
The plane of the figure represents horizontal plane. The air that goes from the A side of the ball in the figure is dragged by the spin of the ball and its speed increases. The air that goes from the B side of the ball in the figure suffers an opposite drag and its speed decreases. The pressure of air is reduced on the A side and is increased on the B side as required by the Bernoulli’s theorem. As a result a net force F acts on the ball from the B side to the A side due to this pressure difference. This force causes the deviation of the plane of motion.