functions-and-graphs-4

Functions:

Sketch of curve y = sec(sec^-1x):

Domain R - (-1, 1)
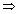
& range y = x   y R - (-1, 1)
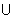
y = sec(sec^-1x) = x, only when x (-, -1) (1, )




Sketch of y = cot(cot^-1x):

Domain R - (-1, 1)
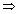
& range y = x   y = R
We should sketch
y = cot(cot^-1x) = x v x R




Sketch of y = sin^-1: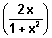

Domain [-1, 1] v x R
& range y
b/c   y = sin^-1
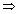
    y




Dumb Question: How domain is [-1, 1] v x R ?

Ans:    i.e.   
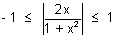
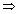
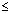
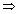
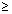
   2|x| 1 + x²   |x|² - 2|x| + 1 0
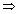
   (|x| - 1)² 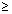0
   x R
Let   x = tan
      
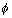
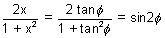
y = sin^-1(sin2) =
                      
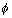
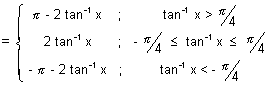
This curve has sharp edge at x = ± 1
So, not differentiable at x = ± 1


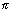
Dumb Question: How does y = - 2 for 2 >

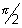
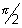
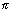
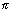
Ans: Since our y . So, if 2 > to make it we substract it from - 2 because sin( - 2) = sin2


Sketch of y = cos^-1

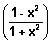
For domain

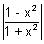
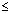
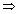
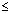
1   |1 - x²| 1 + x²
Which is true for all n; as 1 + x² > 1 - x²    x R
domain [-1, 1]
For range
    y =cos^-1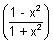
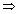
y (0, )
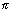
Let x = tan
y = cos^-1 = cos^-1(cos2)
  




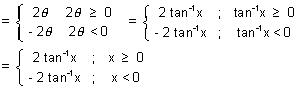Dumb Question: How cos^-1(cos2) = - 2 tan^-1x when x < 0 or < 0

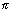
Ans: Range of y [0, ]. So, when < 0 or x < 0
Then we have to make it b/w [0, ]
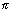
So, cos^-1(cos2) = - 2 when < 0
because

cos(- 2) = cos2

This curve has sharp edge at x = 0. So, not differentiable at x = 0.


Sketch of y = tan^-1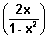

For domain
R     except   1 - x² = 0
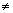
i.e.   x ± 1
or   x R - {1, -1}
domain R



Range   y = tan^-1
as        y = tan^-1    y
Let   x = tan
y = tan^-1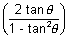 = tan^-1(tan2) =
  


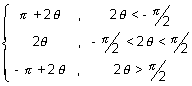
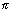
Dumb Question: Why y = tan^-1(tan2) = - + 2 tan^-1x, for > ?

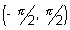
Ans: Range of y   & when >    So, 2 > .
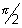
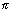
To make it within range we substract So, it comes under range.
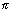
Because of tan(- + 2) = tan2, this can be done.
This curve is neither continous nor differentiable at x = {-1, 1}


Sketch of curve y = tan^-1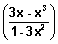

For domain,
    y = tan^-1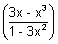
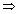
x r except     1 - 3x² = 0    x ±
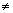
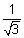
  x R - {± }
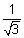
domain R
For range
    y = tan^-1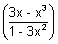
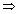
y
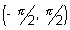
Let   x = tan
y = tan^-1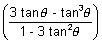 = tan^-1(tan3) =
  




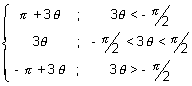
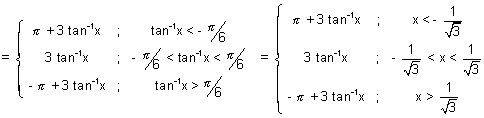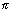
Dumb Question: Why tan^-1(tan3) = + 3    if    < - ?

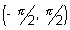
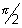
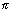
Ans: Range of tan^-1(tan3) is but if < -   So, 3 < -   So, to make it within given range. We add to it.
and in III^rd quadrant.
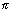
tan( + 3) = tan3
So, this is done.
This curve is neither cont. nor differentiable at x = ± .


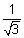
Sketch of   y = sin^-1(3x - 4x³):

For domain
    y = sin^-1(3x - 4x³)
x [-1, 1]
For range
y = sin^-1(3x - 4x³)   y
Let x = sin
y = sin^-1(sin3) =
  


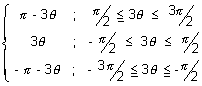
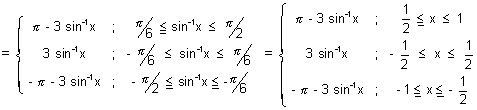
This curve has sharp edge at x = ± 1/2   so, not differentiable at x = ± 1/2.