Gravitation -4

(b) New potential energy = U_f = -
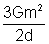
Work done in formation of new style = +
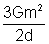
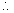
Work done by external force = -
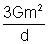
(a) Potential Energy of Complete System = U₁₂ + U₂₃ + U₃₁

    

VARIATION IN ACCELERATION DUE TO GRAVITY:
(a) With Altitude, g = g₀(1 - )
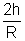
(b) With depth, g = g₀(1 - )

Why ??
With Altitude:
At the surface of the earth       g =
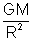
for height 'h' above the surface of the earth,
g' =

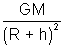
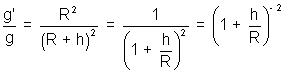
for h << R. Using Binomial approxiation.

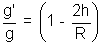
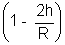
or g' = g     [note: formula valid only for h << R]

With depth:



Suppose body is at depth d assuing density of eargth to remain same throughout.
From Shell's theorem, the body at this depth will only experience the force because of sphere, it is enclosing
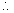
man of that sphere =
    
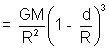
force on unit mass due to it = g
    


Illustration: Calculate the acceleration due to gravity at the surface of Mars, if its man is 1/10 th that of the earth and diameter 1/1.88 that of erth, (g_E = 9.8 m/s²).
Solution: We know g =

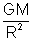
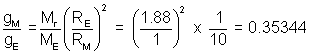
g_M = 0.35344 g_E
    = 0.35 x 9.8
    = 3.48 m/s²

ESCAPE SPEED:
It is the minimum speed with which a body must be projected from the surface of a planet. So that it escapes from the gravitational field of the planet (i.e. it comes to rest at infinity)
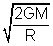
V_e =       V_e = 11.2 km/s

Why ??
Potential energy of the body at surface of planet = -
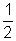
If it is launched of planet = mV_e²
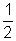
Total mechanical energy of system = mV_e² -
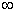
At infinity potential energy = 0    (as is assumed ....)
Kinetic energy = 0    (as body comes to rest hypothetically at infinity
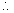
Total mechanical energy now = 0
From conservation of mechanical energy
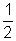
    mV_e² - = 0     or     V_e =
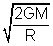
It is important to not that escape speed is independent of the mass and direction of projection of the body.

Illustration: The masses and radii of the earth and the moon are M₁, R₁ and M₂, R₂ respectively. Their centres are at a distance d apart. What is the minimum speed with which a particle of man m should be projected from a point midway between the two centres so as to escape to infinity ?
Solution: Potential energy of m which is midway between M₁ and M₂ is
U = m(v₁ + v₂) = m
  
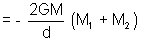
Let v be the requiyed speed, then
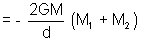
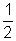
Total mechanical energy + mv²
Total mechanical energy of mechanical energy = 0
From conservation of mechanical energy
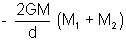
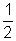
   + mv² = 0
or v =

MOTION OF SATELLITE:
• ORBITAL VELOCITY: