Hyperbola - 3
ILLUSTRATION : 4.
Find the equations of the tangent to the hyperbola 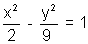 which is perpendicular to the line 3y + x =1
which is perpendicular to the line 3y + x =1
Let the slope of line be m then 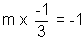
 m = 3
m = 3
& a2 = 2 & b2 = 9
Hence equations of tangents are:
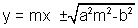
i.e. y = 3x + 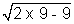
& y = 3x - 
 equations are
equations are
y = 3x + 3
& y = 3x - 3
EQUATIONS OF NORMAL
(a) POINT FORM:
Equations of normal to the hyperbola 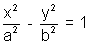 at (x1,y1) is
at (x1,y1) is 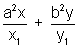 = a2 + b2
= a2 + b2
Why ?
The equations of hyperbola is
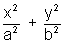 - 1 = 0
- 1 = 0
Differentiating w.r.t.x we get
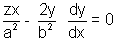


Hence the equation of normal is
(y - y1) = 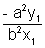 (x - x1)
(x - x1)
 b2x1y - b2x1y1 = - a2y1x + a2x1y1
b2x1y - b2x1y1 = - a2y1x + a2x1y1
Dividing whole equation by x1y1
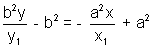

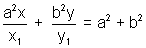 is the required equation
is the required equation
(b) PARAMETRIC FORM:
The equation of normal at (a sec , tan
, tan ) to the hyperbola
) to the hyperbola 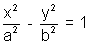 is
is
xa cos + by cot
+ by cot = a2 + b2
= a2 + b2
Why ?
We know that the parametric equations of hyperbola are
x = a sec y = tan
y = tan
Differentiating them we get
dx = a sec tan
tan d
d & dy = bsec2
& dy = bsec2 d
d
Dividing them we get
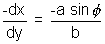
Hence the equation of normal is
(y - b tan ) =
) = 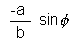 (x - a sec
(x - a sec )
)
 by - b2 tan
by - b2 tan = - ax sin
= - ax sin + a2t an
+ a2t an
 ax sin
ax sin + by = (a2 + b2)tan
+ by = (a2 + b2)tan
Dividing whole equation by tan we get
we get
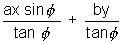 = a2 + b2
= a2 + b2
 as cos
as cos + by cot
+ by cot  = a2 + b2 is required equation.
= a2 + b2 is required equation.
(c) SLOPE FORM:
The equations of the mormals of slope m to the hyperbola 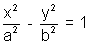 are given by
are given by 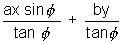
Why ?
We know that the equation of normal at (x1, y1) is 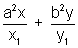 = a2 + b2
= a2 + b2
since m is the slope, then
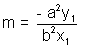

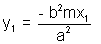
& (x1, y1) lies on 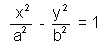


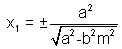
y1 = ±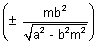
Hence the equation of normal in term of slope is
y - 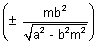 = m
= m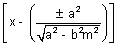
 y = mx ±
y = mx ± 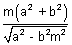 is the required equation of normal in slope form.
is the required equation of normal in slope form.
Illustration : 5.
Find the equation of normal to 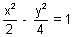 at the poimt whose eccentric angle is
at the poimt whose eccentric angle is  .
.
Ans: We know the equation of normal in terms of eccentric angle is
ax cos + by cot
+ by cot = a2 + b2
= a2 + b2
Here  = 450, a =
= 450, a = 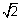 , b = 2
, b = 2
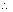 ax cos450 + by cot452 = a2 + b2
ax cos450 + by cot452 = a2 + b2

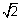 .x.
.x.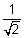 + 2.y.1 = 2 + 4
+ 2.y.1 = 2 + 4
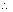 x + 2y = 6 is the required equation.
x + 2y = 6 is the required equation.
EQUATION OF A CHORD BISECTED AT A GIVEN POINT:
The equation of a chord of hyperbola 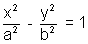 , bisected at (x1, y1) is T = S1 where T =
, bisected at (x1, y1) is T = S1 where T = 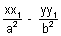 - 1 & S1 =
- 1 & S1 =  - 1
- 1
i.e. 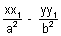 =
= 
Why ?
Let QR be the chord whose mid point is P. Since Q & R lies on hyperbola.

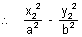 = 1 .............................. (i) & also
= 1 .............................. (i) & also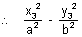 = 1 .............................. (ii)
= 1 .............................. (ii) Subtracting (ii) from (i) we get
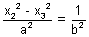 (y22 - y32)
(y22 - y32) 
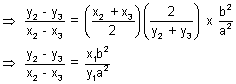
Hence slope of chord joining Q & R is
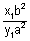
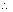 The equation of chord QR is
The equation of chord QR is 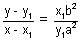
 yy1a2 - y12a2 = xx1b2 - x12b2
yy1a2 - y12a2 = xx1b2 - x12b2Dividing whole equation by a2b2 we get
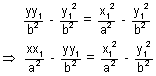

 T = S1
T = S1is the required equation of chord with given mid point.
PAIR OF TANGENTS
The combined equation of pair of tangents drawn from a point (x1, y1) to the hyperbola
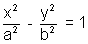 is
is 
i.e. SS1 = T2
where S =
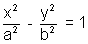 ; S1 =
; S1 = 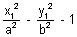 ; T =
; T = 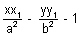
Why ?
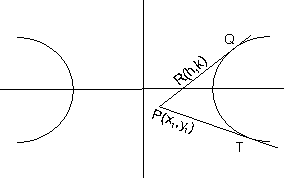
Let R(h, k) be any point on pair of tangents PQ or PT from any external point P(x1, y1) to the hyperbola
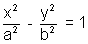 .
. Equation of PR is
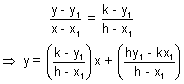
But this line is a tangent to the hyperbola so it must be of the form.
y = mx ±
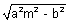
Here c =
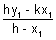 & m =
& m = 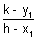
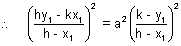 - b2
- b2 (hy1 - kx1)2 = a2(k - y1)2 - b2(h - x1)2
(hy1 - kx1)2 = a2(k - y1)2 - b2(h - x1)2Hence locus of (h, k) is
(xy1 - yx1)2 = a2(y - y1)2 - b2(x - x1)2
 (xy1 -- yx1)2 = - (b2x2 - a2y2) - (b2x12 - a2y12) - 2(a2yy1 - b2xx1)
(xy1 -- yx1)2 = - (b2x2 - a2y2) - (b2x12 - a2y12) - 2(a2yy1 - b2xx1) Dividing whole equation by a2b2 we get
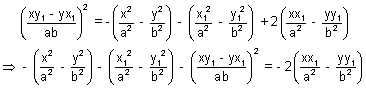
Adding 1 +
 on both sides we get
on both sides we get 
i.e. SS1 = T2