To find limit of a function is an interesting concept where it may be possible that value of the function does not exist at a point but we try to find the value in the neighbourhood of the point. We will talk about this in more detail in the chapter. In the other part of the chapter we will discuss continuity of a function which is closely related to the concept of limits. There are some functions for which graph is continuous while there are others for which this is not the case…
So let us start with the chapter:………………………………
Definition of Limit:
We sometime come across situations when the values of the function ‘f’ for values of ‘x’ near a point ‘c’ lie near a number ‘l’ which is not equal to f (c) or that value lie near no number at all.
Dumb Question:
1) How is it possible that function has different value near point c and at c?
Ans: Let us explain it with help of an example, Consider
Fig (1)
In this function clearly the value of function near 1/2 lies near 1/2 but the value of
function at 1/2 is 1 which is not equal to 1/2.
So, there is need to introduce the notion of limit. A function f is said to tends to a limit l as x tends to ‘a’, if on approaching the point x=a from the values just greater than or just smaller than x=a, f(x) has tendency to move more closer to value l.
Mathematically we write this as
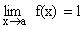Which is equivalent to,
|f(x)-l| < e " x whenever 0 < |x-a| <d, and e and d are sufficiently small positive numbers.
Right and Left hand limits:
Right hand limit:
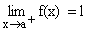If for every numbers e>0 there exists corresponding number d>0 such that for all x satisfying
a < x < a+d
Þ |f(x)-l)| <e
Example: 
Left hand limit:
If for every number e>0 there exists a corresponding d>0 such that for all x satisfying a-d< x < a Þ |f(x)-l|<e
Example: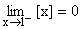 
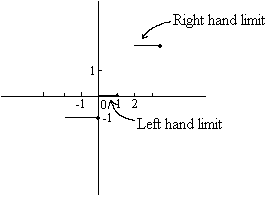
Fig (2)
Note that limit of a function exists at any point if and only if left hand limit is equal to right hand limit at that point.
Illustration 1:
Find the value of 
Where
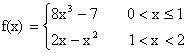
Solution:
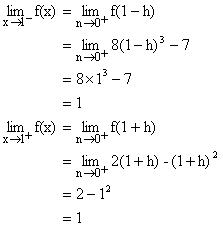
So, right hand limit and left hand limit are equal. Hence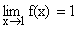 
Discovering infinity:
Infinity (
) is a symbol and not a number it is symbol for something which keeps increasing and passes all limits. Similarly - is symbol of a variable that continuously decrease and passes all limits.
Remember the following points:
1) We cannot plot on paper.
2) + = .
3) - is indeterminate.
4) ´ = .
5) 0 ´ is indeterminate.
6) 
If a is finite.
7) 
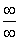, 0,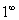 
are all indeterminate.
Dumb Question:
1) It is often said ‘ + a’ where a is finite is . How is this possible?
Ans: Consider like an ocean. Now ‘a’ is like a bucket of water. If you add a bucket of water to ocean will the volume of ocean increase? It will remain ocean. So similarly remains on adding a finite number to it.
Illustration 2:
Find the value of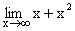 
?
Solution:
= +
=
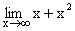\The value of 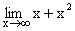= .