Logarithms - 1

Definition:-
We define log as, a^y = x than y = log_ax. in log_ax. both x and a are positive ie. x > 0 and a > 0 and also a 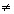1.

Dumb Question:- Why a cannot be 1 ?
Ans:- Suppose a is 1 then let us attempt to y such that y = log₁x.
Now according to definition of log.
1^y = x.
But no matter what power we raise to 1 the answer will be. 1 only so we will never never be able to find y.
Hence a cannot be 1.

Some important formulae:- (Formulaes marked with * are important. This is not be printed)

1. log_aa = 1.
   
2. log_any1 = 0.
   
3. log_ca = log_ba.log_cb.
   Why ?
   Let log_ba = x and log_cb = y
   So, by definition, a = b^x ………………………………. (i)
   b = c^y ……………………………….. (ii)
   Using (i) & (ii) a = c^xy
   Now taking log on both sides.
   log_ca = xy
   log_ba.log_cb.
   
   Illustration - 1.
   Find value of log₂10.log₁₀2 ?
   Using formula 3 we get.
   log₂10.log₁₀2 = log₁₀10
   Now using formula 1 we get
   log₁₀10 = 1
   Hence log₂10.log₁₀2 = 1
   
   
4. log_a(m.n) = log_am + log_an
   Why ?
   Let log_am = x
   log_an = y
   So, m = a^x and n = a^y [Using definition]
   m.n = a^x.a^y = a^{x + y}
   => log_a(mn) = log_aa^{x + y}
                      = x + y
                      = log_am + log_an.