Logarithms - 6

Dumb Question:- Why would R.H.S. be positive only if x > 1 ?
Ans:- R.H.S. is (x - 1)⁷
Now suppose if x < 1 then we have (-ve no) odd number.
Now let us explain this with an example say (- 2)⁷ = - 128 which is - ve.
So, clearly R.H.S. will be +ve only if x > 1.

HARD
Q1. If n is a natural no such that n = and P₁, P₂,.....P_K are distinct primes then show that logn > Klog2.
Ans:- Since n is a natural no and P₁, P₂ ......... P_K are prime nos, so, a₁, a₂ ....... a_K have to be natural nos.
So, a_i ...... 1 for ie. 1, 2, ............. K
Now logn = a₁logP₁ + a₂logP₂ + .................. + a_K logP_K
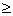
logP₁ + logP₂ + ..................... + logP_n
Now, since P₁, P₂ .................... P_K are distinct primes.
So, P_i 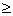2 for i.e. 1, 2, ................. K.
So, logP_i > log2
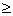
Hence logn log2 + log2 + .................... + log2 = K log2
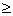
Hence logn K log2

Dumb Questions:- Why a₁, a₂, .................... a_K are natural no.
Ans:- Suppose a₁, ........... a_K - ve no.
than we will have a denominator will not be able to cancel with numerator because the prime nos do not cancel each other. Also if the a_i will be an irrational no so again n cannot be natural no.

Q2. Prove that

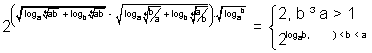Ans:- Since
                                                        =
                                                        =
                                                        =
                                                        =
&

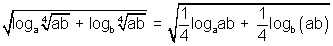
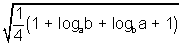
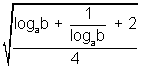
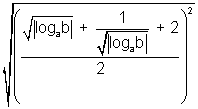
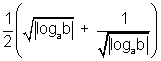
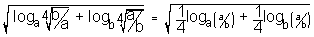
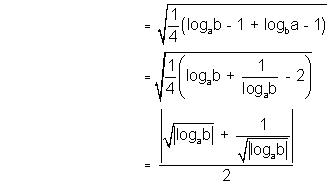
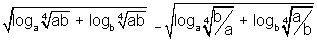
So, = R (say)
So, R =

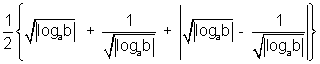
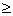
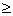
Case I: If b a 1
then P =
           =
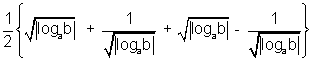
So, = 2¹ = 2

Case II: If 1 < b < a
then P =
           =


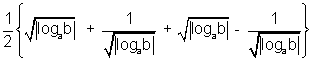
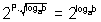Keywords
1. Log
2. Logarithm
3. Exponential