Progression - 2

Illustration 5:

If x = 1+ a+ a²+a³+ --------+¥ and y = 1+b+b²+ b³+ ------ +¥ Show that,

 

Where 0<a<1 and 0<b<1.

Solution:

Given x = 1+ a+ a²+a³+ --------+¥

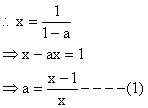
y = 1+b+b²+ b³ ------ +¥

Since 0<a<1, 0<b<1

\ 0<ab<1.

Now  

 

Arithmetic Geometric Series (A.G.P):
Suppose a₁, a₂, a₃, ------- a_n be an A.P and b₁, b₂, b₃, ------- b_n is a G.P Then the sequence a₁b₁, a₂b₂, -------- a_nb_n is A.G.P.

A.G.P is of form

Where clearly

1) Sum of n terms of an A.G.P

Why?

Let

Now if we multiply the series by r then.

So on substraction,

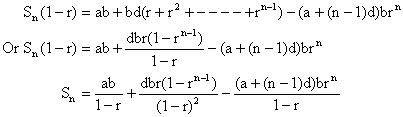
2) Sum of infinite series S_¥.

Why?

If |r|<1 then

So,

Illustration 6:

If sum to infinity of the series then find x.

Solution:

We know that

Here a=1, b=1, r=x, d = 3

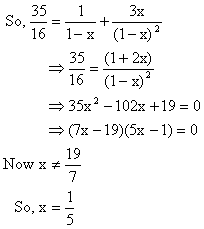
Dumb Question:

1)      Why ?

Ans: For infinite series to be summable |x| needs to be less than 1 hence

 

 

Harmonic Progression (H .P):
The sequence a₁, a₂, ------ a_n is said to be a H.P if is an A.P.

The n^th term of a H.P (t_n) is given by .

 

 

Harmonic Means (H .M):

If H₁, H₂, H₃-------- H_n be n H.M’s between a and b then a, H₁, H₂, H₃-------- H_n, b is a H.P.

This means is a A.P.

And hence

Note:

1)      If a₁, a₂, a₃---------a_n are n non-zero numbers then H.M(H) of these number is given by

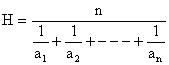
2)      If a, b, c are in H.P then

Why?

a, b, c are in H.P so, are in A.P

And hence

Illustration 7:

If the (m+1)^th, (n+1)^th and (r+1)^th term of an A.P are in G.P m, n, r are in H.P, Show that ratio of the common difference to the first term in the A.P is (-2/n).

Solution:

Let ‘a’ be the first term and ‘d’ be common difference of the A.P. Let x, y, z be the (m+1)^th, (n+1)^th and (r+1)^th term of the A.P then x = a+md, y = a+nd, z = a+rd. Since x, y, z are in G.P.

\ y² = xz i.e. (a+nd)² = (a+rd) (a+md)

Now m, n, r in H.P

 

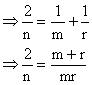
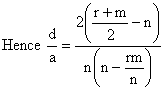
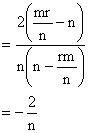
Some Important Theorems:

If A, G, H are respectively AM, GM, HM between two positive unequal quantities then.

1)      A>G>H

Why?

First of all let us Prove A>G.

The two numbers be x, y.

So to prove

Hence A>G -------- (1)

Now Let us Prove G>H

Again

Combining (1) and (2) we get A>G>H.

Why G²=AH?

Let x, y be two numbers.

So,

Hence

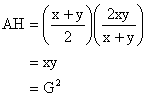
Illustration 8:

If a, b, c, d, be four distinct positive quantities in H.P then show that a+d>b+c.

Solution:

a, b, c, d are in H.P

Then A.M > H.M

For first three terms

Þ a+c>2b ------ (1)

And for last three terms

Þ b+d > 2c ------ (2)

From (1) and (2)

a+c+b+d > 2b+2c

Þ a+d > b+c.