SHM -4

Here
Generalising the result :

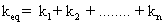
Hence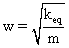
Combining of SHM’s :
The resultant motion of a particle, if 2 forces which produce SHM are applied over it, is a combination of the two SHM’s.
The two SHM’s are then combined using the theory of vectors.
If SHM I :
SHM II :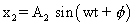
then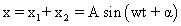
where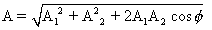
And then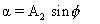
Derivation :

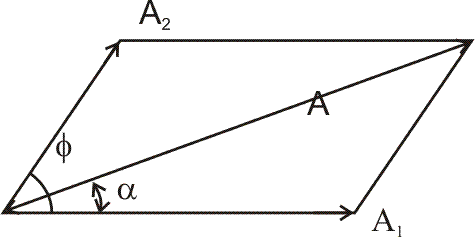
The two SHM’s have a phase diffrences . So this can be treated as the difference in ‘angle’ between the two SHM with magnitude rule..
So by parellelagram rule,
= Resultant vector such that

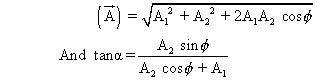
# Illustration : Find the displacement equation of SHM by combining

Solution : Let the resultant equation be :

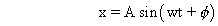
then,

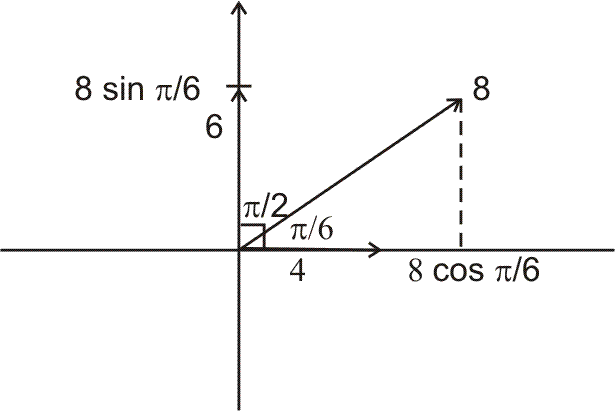
Resultant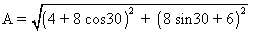


.
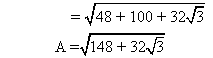
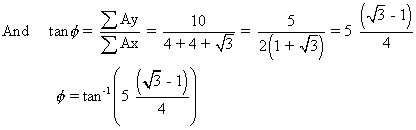
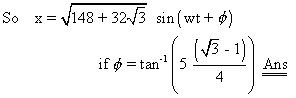Ans. Question :- The Potential energy of a particle ascillating on x axis is where 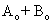are constant.
The total mechanical energy of particle is 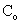joules.
Here 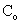is also in joules while x is metres.
Is the motion SHM ? Where is its mean particle?
Solution :-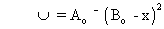

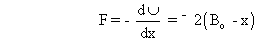
Changing 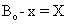{shifting of coordinate axis}
Force 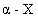so motion is a SHM.
At Mean position Potential energy = minimum and Force = 0
So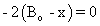
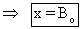Mean position. Ans.
Question - 2. Two particle move parellel to x axis about origin with same amplitude and frequency. At a certain instant, they both are found at a distance A/n from origin (y>1) and they are on opposite sides of the origin. Their velocities are found to be in same direction. Find the phase diference between two ?
Solution :- The problem becomes very simple if we represent both SHM’s on a circle.


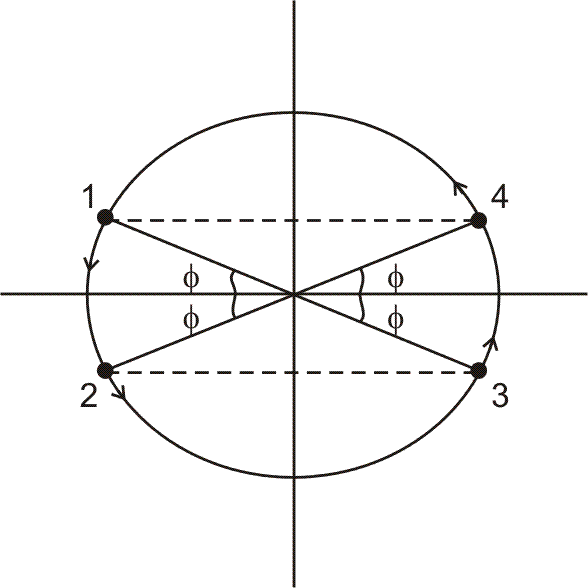
The particle positions are 1/2/3/4
But as they are an opposite sides, and their velocity in same directin so either 1 and 2 or 3 and 4. The phase difference will b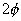e where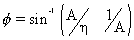
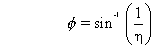Ans.
Question - 3. A particle executes SHM with w = 3.4 and amplitude 2m. Find (a) time period (b) Maximum speed (c) Maximum accelaration (d) Speed where displacement is .5m from mean position.
Solution :- Let SHM be
(a) Time period =
(b) Max Speed = v = 2w = 6.28 m/s.
(c) Max Accelaration =
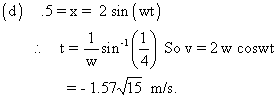Ans.
Question - 4. The pulley of mass = as shown has a moment of Inert = 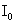. The spring has a spring constant 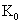. Find the time period of ascillation of its centre of mass ? [string slip over pulley]
Solution :-


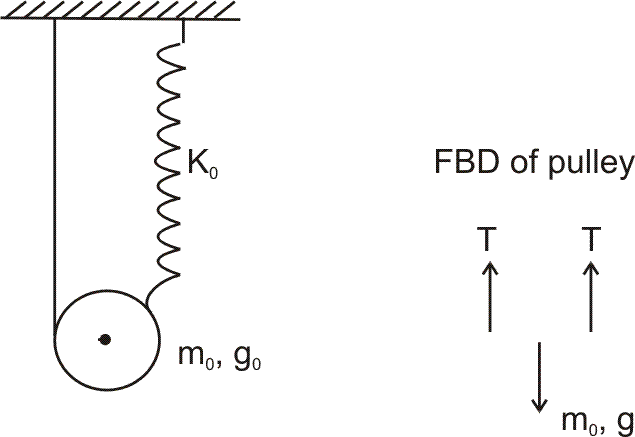
At equilibrium :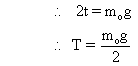
Now let initial extension in spring =

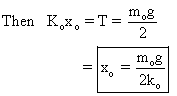
Now we appoach the problem using Energy method :
Suppose centre of mass of pulley goes down by ‘x’. the spring extends further by ‘2x’. Energy of system.

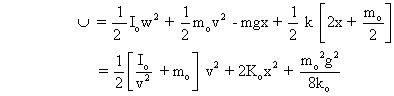
Now 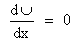So


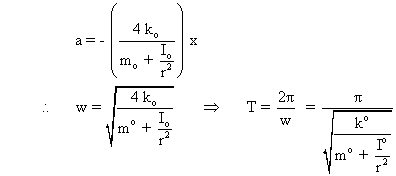
Question - 5. A simple pendulum is susoended from the acting of a car which is moving down the inclined plane with constant velocity. The inclmation angle is Find time period of pendulum ?
Solution :-

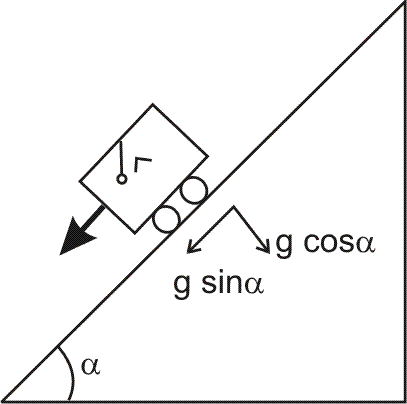
For the pendulum bob;
The 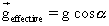in a direction perpendicular to inclined plane ! So we just have to replace the 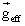instead of 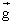in formula for time period o a simple pendulum.

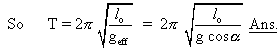
Question - 6. A regid body is suspended from a fixed support O. Find the time period of its ascillations if its moment of initia is ‘I’ distance between O and of mass is ’d' and mass is ?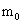
Solution :-

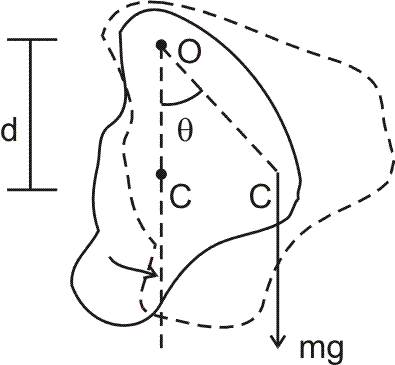
Suppose the body is displaced by small angle 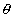. Now torque on body about O is

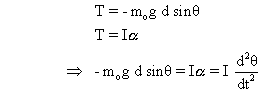
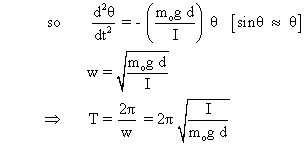
• This result can be remembered as such.